Happy Holidays!

I have received a few holiday gifts I'd like to share with you:

1) A beautiful video from Ken Fan at Girls Angle



The program Ken used to make the video above is a free open source 3D creation suite you can use too. Available at www.blender.org

2) A pointer from Melissa Smith of the Ithaca Math Circle to a great article by Nate and Sam Cornell. Nate is a psychologist at Williams College and Sam is a writer in San Francisco. Their article really accorded with decades of experience for me as a lifelong educator who continues to love her own learning challenges.

Here is a link to the article:
A Really Hard Test Really Helps Learning: Challenging tests and falling short may be hard on the ego, but they can do more than mere studying for eventually getting it right

Here's an excerpt that especially struck Melissa and resonated well with me also:

Both studies independently indicate a striking fact. We tend to assume that the best way to consume and remember information is through the application of rigorous, extended study. What we fail to see, however, is that the process of trying to work through a problem to which we don’t know the answer focuses our attention on it in a way that simply studying it does not. The desire to get the answer right, and the frustration of failure, is partly to account.

But there’s another element as well. When we struggle to learn something, and fail, the moment we finally get the answer it imprints itself more deeply on our mind than it would have had struggle and failure not preceded it.

3) Excellent advice from mathematician Lillian Pierce, currently at the Institute for Advanced Study: Try again! Fail again! Fail better!

4) A random factaroony from Number Gossip: 2011 is the first odious prime number we've had since 1999. For more about "odious" and "evil" numbers, see here.

5) And one last present (especially nice during those bleak and gloomy times when it feels like winter may never end ... or when it feels that a problem may never get solved.) A hat tip to the Metroplex Math Circle for calling my attention to this enchanting video. Double-click on the video for a bigger view to see the whole picture.



For more about the mathematics behind this video, see this website.

And remember ... just as sometimes the most unpromising-looking larvae can turn into the most exquisite and magical dragonflies, so too can the most hopeless looking math problems turn into the most awesome aha! experiences.

May 2011 be filled with many wonderful problems and discoveries! And many wonderful mathematical friends with whom to share them!

AMC8 Congratulations!

Congratulations to all the Albany Area middle school students who embraced the challenge of the AMC8 with such enthusiasm this year! Congratulations as well to our high school students who have mentored many of them.

Honor Roll (scores of 18 to 21)

18 Alex Cao Shaker JHS
18 Alex Wei Van Antwerp MS
20 Patrick Chi Iroquois MS
20 Gideon Schmidt Iroquois MS
21 Shreya Arora Iroquois MS
21 Andrei Akhmetov Van Antwerp MS
21 Alicia Chen Farnsworth MS
21 Zach Benson Hebrew Academy of the Capital District
21 Sean Setzen Hebrew Academy of the Capital District


Honor Roll of Distinction (scores of 22 to 25)

23 Gili Rusak Shaker JHS
24 Philip Sun Acadia MS
24 William Wang Farnsworth MS
25 Ziqing (Bill) Dong Farnsworth MS

We will post additional honor scores here as we receive information about them.

Bill Dong's perfect paper of 25 is extremely unusual--there have been many years when there are no 25's anywhere in the entire state. It is all too easy for even the strongest students to make mistakes on a test like the AMC8, as many of our veteran high school students and alumni can tell you!

Bill joins a very rarefied group of students--Albany Area Math Circle's only previous perfect scorers on the AMC8 have been Raju Krishnamoorthy (1999), Drew Besse (2001), Schuyler Smith (2006), and Matthew Babbitt (2007).

Bill will also receive the AMC8 high scorer in the state award, as did Raju, Drew, Schuyler, and Matthew, as well as Andrew Ardito and Dave Bieber.

Students who would like to prepare for next year can find lots of old AMC8, AMC10, and AMC12 test problems, along with hints and solutions free and online at this webpage.

Fall Middle School Math Meets

Thanks to everyone who contributed to the success of our inaugural season of Fall Math Meets! Watching you smile and make new mathematical friends and share cool math as you talked over the problems together after the contest is what makes it all worthwhile for us volunteer advisors.

There are many ways to measure success--we recognize a few of them here, but the ultimate measure of success is whether you continued thinking about and talking over the new ideas you learned afterwards.

October High Scorers:

8 points:
Zachary Benson, Sophie Rich, Helen Yuan

9 points:
Emily Honen, Jeffrey Shen, Cathy Shi, Max Thomas

10 points:
Bill Dong, Thomas Glozman, Ben Salem, Gideon Schmidt, Philip Sun, William Wang

"Whole is more than the sum of the parts" team award:
Adam, Emily, Jerry, Max


November High Scorers:

6 points:
Zachary Benson, Gwenda Law, Michelle Yu

9 points:
Alex Cao, Patrick Chi, Jerry Qu, Gideon Schmidt, William Wang

10 points:
Alicia Chen, Bill Dong, Philip Sun, Alex Wei

High scoring newcomer award:
Samuel Enriquez, Rafi Nizam, Jason Tang, Michael Zhu

"Whole is more than the sum of the parts" team award:
Jason, Mike, Zach


December High Scorers:

7 points:
Alex Cao, Thomas Glozman, Michelle Yu

8 points:
Bill Dong, Jerry Qu, Gideon Schmidt

10 points:
Alicia Chen, Philip Sun, William Wang, Alex Wei

High scoring newcomer award:
Sean Dory

"Whole is more than the sum of the parts" team award:
Daniel, Nabihah, Swetha, Thomas, Yang

In memoriam: Joel Brainard

Our thoughts and hearts go out to Joel's daughter, Katherine Brainard, a founding member of our math circle, and to Joel's wife, JC Glendinning, an important driving force behind the beginnings of our math circle in 2001, and to their entire family.

Joel Pennington Brainard, 71, died peacefully on December 11, 2010, at NY Presbyterian Hospital in New York, NY. He is survived by his wife Jane Carol Glendinning; their children, Junior, 29, Katherine, 25, and Scott, 23; and his brothers Charles and William. A graduate of Oberlin College, Joel served in the Peace Corp in the Ivory Coast and later taught mathematics at Talladega College in Alabama. With advanced degrees from MIT and Cornell University, Joel was an engineer on energy conservation projects at Brookhaven National Laboratories on Long Island and was a consultant on public utility issues for the Vermont Low-Income Advocacy Council through Vermont Legal Aid in Burlington. His many colleagues remember him fondly for his more than 22 years of service as an economist and manager in the Office of Research at the NYS Public Service Commission. Joel was an extraordinary man whose thought, wit and kindness touched the lives of all who knew him. Family and friends remember his joy for life with deep affection. His energy and enthusiasm permeated his life, particularly his ingenious solutions of problems, both large and small. From reducing distortions in the pricing of the electrical grid to his unique approaches to car repairs, home construction, and even ski boot insulation and golf cart-to-ATV modification, Joel provided an inspiring example and will be greatly missed. In lieu of flowers, the family requests that gifts be made in Joel's memory to the Robert C. Parker School, which Joel helped found 20 years ago, or the Scleroderma Foundation, an institution which works to find treatments for an autoimmune condition that Joel fought creatively for many years. A Memorial Service will be held on Saturday, December 18th at 11:00 am at the Robert C. Parker School, 4254 West Sand Lake Rd, Wynantskill, NY.

The biggest Evil Abundant Number submitted at today's Middle School Math Meet?

The teams at our middle school math meet today submitted the following numbers as candidates for the "biggest evil abundant number you can find."

24
720
111100
and

drumroll please....


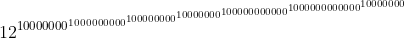
And the winner is.....well, that's not so clear. We'll discuss it below. We'll also tell you what evil numbers and abundant numbers are.

But, first, a few important words of thanks!

We had a GREAT Middle School Math Meet today! Thanks very much to Felix Sun, Qun Lu, and the Principal of the CCC Chinese School Jianzhong Tang for making arrangements to host our December Middle School Math Meet at Shaker Junior High this afternoon. Thanks as well to Felix's mother, Le Xu, for organizing refreshments!

Thanks to UAlbany Professor Rita Biswas, Hebrew Academy math teacher Alexandra Schmidt, and Doyle Middle School math teacher Nancy Smith for helping to run the Math Meet. Thanks as well to our outstanding high school student coaches: Felix Sun (Shenendahoah High School), Zubin Mukerjee (Guilderland HS), Cecilia Holodak and Flora Mao (Niskayuna HS), Simran Rastogi and Gili Rusak (Shaker).

Thanks to all the students who came and worked enthusiastically on the problems.

Thanks to George Reuter of mathmeets.com, who did a great job of writing more great contest problems for the December math meet. We can't discuss those questions yet, since other teams may still be taking that contest.

Okay, so back to this evil and abundant question, which we CAN discuss, since I just created it as a little supplementary challenge to fill in the bits and pieces of waiting time during the meet. It turned out to be way more interesting than I had realized!

We began the Math Meet by discussing the "number of the day: 12." (Why, because it is December 12, or 12/12, of course!)



Like all numbers, 12 has many interesting properties. We focused on two of those properties today, which are highlighted in the Tagxedo-produced graphic above.

Twelve is an "evil number", which means that it has an even number of ones in its binary expansion, i.e., 1100_{base 2} = 1*8 + 1*4 + 0*2 + 0*1 = 12.

Twelve is also an "abundant number," because 12 is less than the sum of its proper factors, i.e., 12 < 14 = 1+2+3+4+6. In fact, it is the smallest abundant number, and therefore, of course, it is also the smallest evil abundant number, as well.

Is there a largest evil abundant number?

Some students noted that doubling an evil number always gives you another evil number! (Why?) What if you tripled an evil number? Or multiplied your evil number by other integers? What if you add two evil numbers? What if you raise an evil number to a power? Do you always get another evil number?

(By the way, you could ask the same questions about "odious numbers," which are the opposite of evil numbers--they have an odd number of ones in their binary expansions.)

Other students noted that doubling an abundant number always gives you another abundant number! (Why?) What if you tripled an abundant number? Or multiplied your abundant number by other integers?

Again, you could ask the same questions about perfect numbers, or deficient numbers.

More interesting questions: can a power of two ever be an evil number? Why or why not? Can a power or two ever be an abundant number? What about powers of three?

All great questions to think about!

Now back to judging the entries submitted in the contest.

24 is clearly evil (binary representation is 11000) and abundant (its proper factors are 1,2,3,4,6,8,12, which sum to more than 24.)

720 is also evil (binary representation is 1011010000) and abundant (its proper factors are 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 16, 18, 20, 24, 30, 36, 40, 45, 48, 60, 72, 80, 90, 120, 144, 180, 240, 360).

111100 turns out to be trickier. It is not clear whether it was intended to be interpreted as a binary number, in which case it would obviously be evil. If it was intended to be interpreted as a base-10 number, then it's actually not evil, because the binary representation of 111100_{base 10} is 11011000111111100. So the only way this number is a candidate is if we interpret 111100 as intended to be binary, in which case it is only equivalent to decimal 60. Now, 60 is clearly abundant, but it is less than 720.

Now, for that humongous number with all the concatenated exponents. I can tell you it is abundant, for sure, but I have no idea if it is evil or not. I am not convinced that the team that submitted it is sure whether it is evil or not, but if they can come up with a convincing proof that it is evil, I am willing to listen. Thinking systematically about some of the questions I raised above may help you sort out what is going on with your--very interesting--number. You may also want to consult the following book, which introduced the concepts of evil and odious numbers: Winning Ways for Your Mathematical Plays Volume 3, by Elwyn R. Berlekamp, John Horton Conway, and Richard K. Guy.

In the meantime, until such time as the orange team can demonstrate their candidate number is evil, 720 is the winner among the numbers submitted today.

Congratulations to the Green Team: Sean, Jason, Gideon, Frank, and Aaron. Your entry of 720 is the largest confirmed evil abundant number of those submitted today.

And, everyone, keep on thinking about this problem! Can you come up with a formula for an arbitrarily large evil abundant number that you can PROVE will work?